The Golden Mean Spiral Re-discovered
Abstract:
In this paper, we explain by pure geometrical analysis the existence of manifold types of golden mean spirals. This is in opposition to the common belief that there is only one golden spiral, discovered by Leonardo Fibonacci around the year 1200. We will show that there are different ways to construct golden spirals and how these different systems are geometrically interrelated. Further, we will explain how these principles can apply to logarithmic/fractal antenna design.
Geometrical analysis of the golden mean spiral:
To begin, we show here the image of the classic golden mean spiral, which most people probably will have seen at some point.
This is the classic golden ratio spiral, and as the mind so created it, is the mind which understands it from a particular perspective. The paradigm from which we see the world is based on our rectilinear conception. When applied to the golden rectangle, which when rotated at 90 degrees, scales up or down the golden ratio, we there see the generation of the well known golden mean spiral.
But if we progress deeper in our geometrical analysis, another way to look, another viewpoint of geometrical perspective is: rather than simply use the rectangle we use the complete circles that compose the arcs within the rectangles, and divide every cycle by 4 sections. We then ignore the rectangles and we proceed to rotate the circles every 90 degrees, where every circle become golden ratio to the next consecutively.
In this perspective we could argue that the golden rectangles are, or could simply be, a ‘geometrical curiosity’, and the circles are the real way of creating the spiral. Or better, they best express the process by which they are generated. Effectively every spiral is made of a multitude of arcs.
Here we see the overlap of the two systems:
This very idea of using circles occurred to me like a flash, a vision I had in my younger age. I imagined a circle in static equilibrium and then seeing the same circle rotating within itself, becoming smaller and smaller, producing a spiral geometry. At that time I create a spiral but I had no idea about the connection with the golden mean spiral.
Interestingly, in the process of writing this article I came across this quote:
“The Spiral is a spiritualized circle, in the spiral form, the circle uncoiled, has ceased to be vicious, it has been set free” -'Vladimir Nabokov
Spirit - s-phi-ral
After many years, I have become very interested in the research of geometry. I found the relationship the golden spiral has with the spiral I created in my youth. Very quickly I realized that other iterations were possible, as it was in the first system I imagined which did not have a specific number of axes or particular proportions. I saw it at that time as fully flexible and dynamic system.
So I envisioned that we are not held to limited axes of symmetry when any number or ratio was possible. This place where I come from helped me to see out of the box, quite literally.
In the next illustrations we will show golden spirals of different numbers. Geometrically speaking no one could argue that they don't contain the golden mean ratio, and that in strict geometrical sense these are golden ratio spirals.
Phi spirals of 6
phi spiral of 7
Here also what we have called the phi-ve golden mean spiral based on 5 which as we know contains golden ratio like any other number. This spiral can be seen as the queen of the golden spirals.
Phi spirals of 6
Another co-incidence here is the work of my father, the renowned Austrian sculpture Adolfo Schlosser. By pure feeling he traced the queen golden spiral with a piece of metal and created a sculpture that represented it many years before this was discovered.
.
Moreover, we would like to show a property that also demonstrates how the golden proportion is contained in the different numbers of the different types of golden spirals and how they relate to the specific geometrical characteristics given by the particular number in which every spiral is generated..
A golden spiral of 5 needs to be scale by the golden ratio 5 times till it meets its interior. A golden spiral of 4 needs to be scaled 4 times to create the same result, of self similarity, shown as the iteration of the same pattern found at different scales.
These images visually and geometrically demonstrate the very definition of what a spiral is, as the simplest possible geometric fractal. They represent the essence of fractality, as one part contains the whole. This concept also exists in projective geometry, the space and counter space of the Steiner cosmogony, the ‘as above so below’ axiom of the Hermetic principles. From the macrocosm to microcosm this property is an essential principle of creation. And we state here that space is not 3-dimensional in nature, as most people believe, but rather is one dimension, space, with fractal, projective and holographic properties. It is our natural experience of space which provides the three dimensions as a coordinate system of reference, projecting our inner 3-fold nature outwardly.
Also, forgive us to intentionally omit any mathematical conjecture, as we use only geometrical visual descriptions. We follow very much the philosophy of Victor Schauberger:
‘We need no science of formulae, but a science of forms.’
As well as John Ruskin, who when asked ‘What is Life?’ responded simply:
‘Always stand by Form against Force.’
Also you may notice that we didn't just lay out this spiral in its golden circles. Well, this is also a question of geometric viewpoint. We can use one system or another to create or describe the spiral, the system is an abstraction. It is a mind-made instrument one may utilise to describe the inherent properties of the system or use it to describe the spiral, but still, we use a chosen system. You can't swim in ‘word water’ as Alan Watts once said. Now the circle methodology has become the pure idea principal in which the spiral creation is described, realising these two systems are parallel or synonymous. In this case, the so called logarithmic system, as a geometrical way to draw spirals, is a methodology that produces more precise results, and is what we opt to use.
This may sound contradictory to you, but stay with us and we will show you geometrically what is happening here.
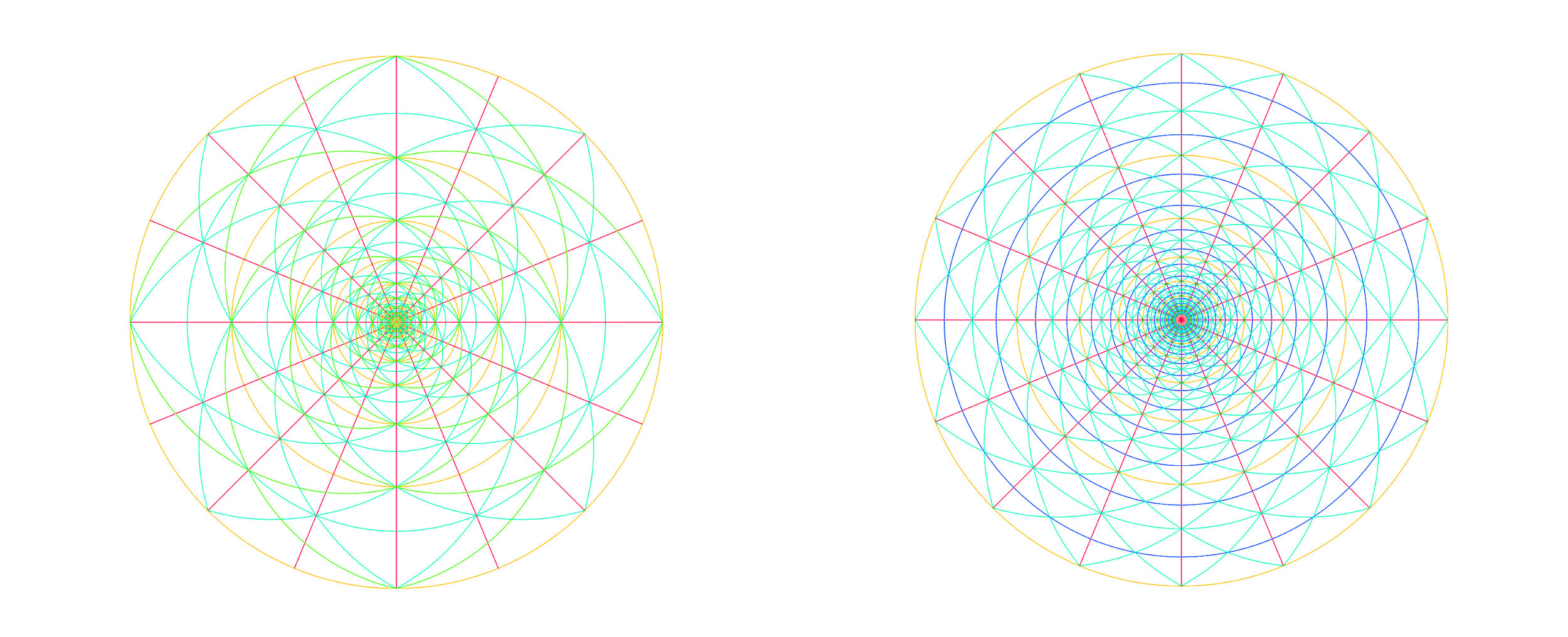
Considering the way all this came into our comprehension is very informative to also cognise this process. This occurs from the idea that when we lay the spiral in the mandala array of different numbers, related to the specific golden spiral number in which it was produced, the crossover of the spirals meet at exact golden ratio concentric circles. This happens only, strictly in this manner, when we use the same number of array spirals in which the spiral was created, again showing the golden mean essence contained within it.
Once we trace radially the corresponding lines we find to our surprise that we are using the classic way of drawing logarithmic spirals!
Having found the parallel quality of these 2 systems .now we can generate greater resolution in the way we approach the utilization of this system to create and generate more exact and precise spirals.
Moreover, we can find other points of intersection that can be seen as subharmonics of the golden ratio. This is found by octaving the number of spirals. For example, a spiral of 4 can create 8,16, 24, generating more circles that have a circular concentric pattern compose of circles , which has the property to find itself at different scales (in the same way the spirals do ), the same iterative pattern appears in specific proportions inward or upwards, as within so without, one part contain contains the whole, in form and proportional relationships, being the specific qualitative nature of fractal systems.
These previous concepts have also a relation to formative motion of energy, and the design of harmonic technologies. In other words, the creation of motion and the motion of creation, as the process to begin to cognise the manifestation of form. Another fascinating idea that correlates to this, is the phenomenon that when this principle is used in fractal or logarithmic antenna design, a holographic field effect will extend beyond the physical antenna. This creates a virtual projection of this recursive energetic pattern, just like heterodyning frequencies will keep generating new derived frequencies that will add and multiply into infinity. However, when dealing with physical forms as per the ideas in this discussion, we can create energetic structures in space, which may not be producing the same effects, than simply mixing frequencies.
This principle is in operation at our logarithmic fractal antenna designs, and is also very active in what we term the BioArc Disc in the image below (designed by J. Schlosser and T.J Brown) and its predecessor the Multiple Wave Oscillator (MWO) invented by Georges Lakhovsky. This same energetic design language can be used in the creation of architecture, as well as all forms of practical applications to the design of our self-created environments and the evolution of beneficial technologies.